Baumol model
The Baumol model, also known as the Baumol-Allais-Tobin (BAT) model, is a cash management model.In 1952, William Baumol presented the idea of managing the surplus of funds through the optimal use of stock supply quantities. He came to the conclusion that money can also be treated as a specific type of stock, one that is necessary when doing business. When we talk about cash optimization and their balances, there is a clear analogy between cash and materials. When we compare cash management and inventory management, it results from the fact that cash surpluses are kept in enterprises as securities, most often they are treasury bills. The Baumol model is based on the economic model of supply size, i.e. Model EOQ (economic order quantity). The main assumptions of the BAT model include:
- possible to be forecast and fixed for the entire period, the demand for cash,
- constant and predictable inflow of cash,
- fixed interest rate throughout the period when investing in securities,
- rhythmic cash receipts,
- instant cash transfers,
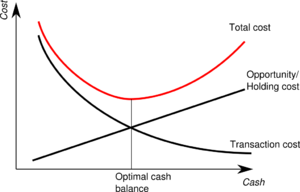
Looking at the above assumptions, one can draw conclusions that cash is consumed in a steady manner. At the moment when they reach the minimum level, the one equal to 0, then the equivalence of cash is converted into cash in such a height as to reach the maximum level. Then the cycle repeats.
The task of the Baumol model is to show the bottom margin of security and at the moment when the current funds approach is approaching this point, then the sale of Treasury bills or other securities is completed in order to supplement the funds. In the Baumol model, the optimal cash level is calculated as follows:
Insert non-formatted text here
C =
Where:
C-optimal cash level
T-demand for cash over the entire period considered (year)
F-fixed costs of cash transfer
R-alternative cost of maintaining cash.
This formula comes from the fact that if the level of cash is to be optimal, then the following equality must exist: KA = KT, the alternative cost must equal the transaction costs. These, in turn, are calculated as follows:
KA=
KT=
The Baumol model is used to determine the appropriate level of cash, which will minimize the total transaction costs and alternative costs as a result of maintaining a given level of cash.
Restrictions on the Baumol model
Although the Baumol model is a classic model of cash management, it is difficult to apply it in everyday life. The main limitation is that the company has to use up the stock evenly in order for the model to work. In practice, this is almost impossible. Also, the difficulty is that in the enterprise it is difficult to determine the precise demand for financial resources, also the expenses incurred by the company do not spread equally over the entire period. Another limitation in the application of the model is also a time-varying transaction commission, which can often be negotiated and depends on the size of the transaction and the maturity date.Another difficulty is that the interest rate on the current account is variable over time, as is the yield on treasury bills, which additionally depends on the maturity of separate series.
When planning the optimal level of financial resources, the Baumol Model is a helpful tool, but it has many limitations that reduce its usefulness. The model is based on assumptions that are not realistic for the company, therefore it is not used in the work.
References
- Tavor, T., Gonen, L. D., Weber, M., & Spiegel, U. (2018). The Modified Baumol Equation: Theory and Evidence. Review of European Studies, 10(1), 25.
- Gonen, L. D., Weber, M., Tavor, T., & Spiegel, U. (2017). Holding Cash and Spontaneous Behavior: A Modification of the Baumol Equation. Review of European Studies, 9(1), 209.
- Dequan, Z., & Yunlong, D. (2014). Study on the Effect of Rising Service Employment on Productivity Growth in China: A Test of Baumol’s Model. Journal of Applied Sciences, 14(5), 482-488.