Marginal revenue

Marginal revenue - is the total increase caused by the increase in production by one unit.

$U_{k}={\Delta U_{c} \over \Delta p}$

$U_{k}$ = marginal revenue (MR)

$\Delta U_{k}$ = change in total revenue (TR)

$\Delta p$ = increase in production by one unit

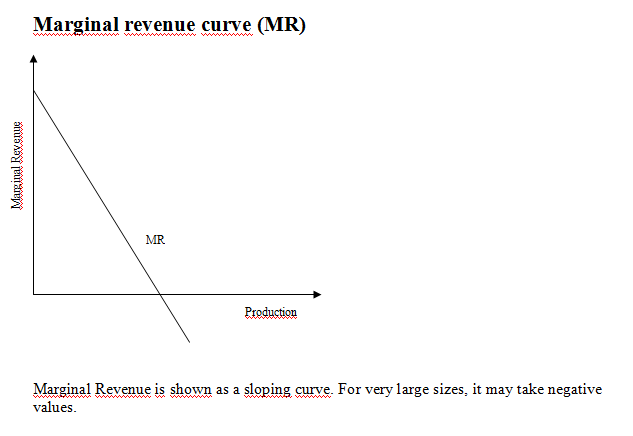
Marginal Revenue is the change in the total revenue depending upon the evolution of the demand curve for a company's product.

An analysis of the marginal revenue combined with the marginal costs associated with the formation of an additional production unit allows you to explore how the increase in production by one unit will affect the profit level. Categories of marginal revenue and marginal cost are used to calculate the production volume that would allow a company to maximize its profits. When the marginal revenue exceeds the marginal costs, production should be increased, but when marginal revenue decreases below marginal cost, production should cease to grow because each additional product unit will reduce the profit level. Profits accrue their maximum gains in production in instances where the marginal cost equals the marginal revenue.

Marginal revenue curve (MR)

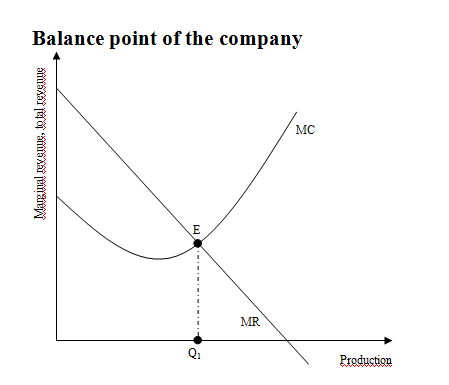
Balance point of the company

$MR$ - line of marginal revenue

$MC$ - marginal cost curve

$E$ - the intersection of MR = MC company' optimum

$Q_{1}$ - ensuring optimum production volume the highest profit

For all points on the left of the $Q_{1}$, the marginal revenue is higher than the marginal cost which means that we can increase production until $Q_{1}$. This is the optimal value. If any profits occur, you should maintain the production volume. In any other case, you should consider suspending or eliminating production. When the level of production is on the right of point Q1, the marginal costs are higher than marginal revenue; I suggest a reduction in production.

Examples of Marginal revenue

• In a perfectly competitive market, the marginal revenue of a firm is equal to the market price. This is because a firm must lower the price of all its units to increase the quantity sold. For example, if the market price for a product is $10, then the marginal revenue for an additional item sold is also $10.
• In a monopoly, the marginal revenue is always lower than the price of the product. This is because a monopolist must lower the price of all its units when it wants to increase the quantity sold. For example, if a monopolist charges $20 for a product, then the marginal revenue for an additional item sold may be $15.
• In an oligopoly market, the marginal revenue of a firm may be different from the market price. This is because the actions of one firm can significantly impact the demand and pricing of other firms in the market. For example, if two firms are selling the same product and one firm lowers its price, then the other firm may have to lower its price as well in order to remain competitive. In this case, the marginal revenue for an additional item sold by the second firm may be lower than the market price.

Advantages of Marginal revenue

Marginal revenue offers a number of advantages, including:

• It allows businesses to assess the profitability of increasing production by a single unit, which helps them make more informed decisions when setting prices.
• It provides a more accurate assessment of the total effect of a price change than simply looking at the average price.
• It helps businesses evaluate the potential impact of changes in the cost of production, as well as the potential effect of changes in demand.
• It also allows businesses to identify potential areas for increasing efficiency and profitability.

Limitations of Marginal revenue

Marginal revenue is an important concept for businesses to understand, however it does have some limitations. These include:

• Not taking into account the costs of production, so it does not show the full economic effect of increasing production.
• It assumes that all units produced are sold, so it does not reflect the reality of a declining demand.
• It does not account for the possibility of competitors entering the market and decreasing the price of the product.
• It assumes that all factors of production remain constant, and ignores the potential impact of changes in technology, labour costs, and other costs.

Other approaches related to Marginal revenue

Marginal revenue is one of the most important concepts in economics, as it helps to understand how to maximize profits. Other approaches related to marginal revenue include*:

• Average Revenue: Average revenue is the total revenue divided by the number of units. It is the average amount received from the sale of each unit of a good or service.
• Marginal Cost: Marginal cost is the change in the total cost caused by the production of an additional unit of a good or service.
• Marginal Profit: Marginal profit is the change in total profit caused by the production of an additional unit of a good or service.
• Marginal Productivity: Marginal productivity is the additional output of a unit of input.

In summary, marginal revenue is an important concept in economics and is related to other approaches such as average revenue, marginal cost, marginal profit, and marginal productivity. These concepts help to understand how to maximize profits and optimize production.

Marginal revenue — recommended articles

Sales price variance — Variable Cost Ratio — Marginal private benefit — Sales mix — Contribution margin ratio — Cross elasticity of demand — Real cost — Baumol model — Cost per unit

References

• Somppi, K. (1985). Salary vs. marginal revenue product under monopsony and competition: The case of professional basketball. Atlantic Economic Journal, 13(3), 50-59.

Author: Maksymiliana Piekarz