Adjusted mean: Difference between revisions
Ceopediabot (talk | contribs) m (→Adjusted mean interpretation: Typos, typos fixed: reasercher → researcher) |
|||
| Line 25: | Line 25: | ||
* [[standard]] deviation, | * [[standard]] deviation, | ||
* and other statistical measures. | * and other statistical measures. | ||
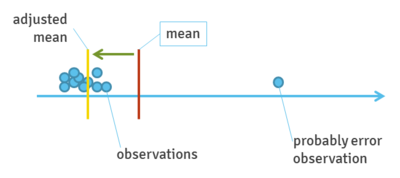
[[File:adjusted_mean.png|400px|right|thumb|Fig.1. Adjusted mean example]] | |||
==Adjusted mean interpretation== | ==Adjusted mean interpretation== | ||
Revision as of 11:54, 31 August 2020
| Adjusted mean |
|---|
| See also |
Adjusted mean - term used in statistics, in statistical analysis. Otherwise called least-squares mean.
Is a mean adjusted for certain data that could lead to misinterpretation. If in the data, far from center there are some values, the mean won't work properly [1]. Adjusted mean is often used in an ANCOVA (Analysis of Covariance) [2].
Adjusted mean usage
For example, try to calculate mean from values: 2, 3, 3, 4, 3, 4, 5, 2, 130. The last value will move average significantly. Mathematically it is correct, but in real life it is probable that 130 is an error or one-time value. Adjusted mean, known also as least-squares mean lets remove such outliers and present [3].
Mean is a measure that can be easily manipulated and thus misinterpreted. It should always be used together with [4]:
- standard deviation,
- and other statistical measures.
Adjusted mean interpretation
An adjusted mean determines an expected result. To precise, it is the predicted mean dependent variable score for studied group of subjects in the situation, when the covariate mean has exactly the same value as the grand covariate mean. The various samples from studies, even when using the method of random sampling, usually cannot provide exactly the same value of covariate mean. Because of that and the fact that covariate is highly related to the dependent variable, the head researcher may consider the reason of that. For example, this situation may be caused by some differences between studied groups. By computing adjusted means, it is possible to find an answer for this question [5].
In a single scientific study there is a high probability of appearance of differences among sample means, due to sampling hesitation. But in the long run the group means will have an equal value [6].
Adjusted means in ANCOVA
In an ANCOVA adjusted Y means are established by subtracting the part of the value form results that are related to or predictable from the covariates. Thanks to this solution it is possible to make a correction of any confound of the covariates or suppress some of the error variance and most importantly, increase the statistical power of conducted testes [7].
Footnotes
References
- Huitema B.E. (2011), The Analysis of Covariance and Alternatives: Statistical Methods for Experiments, Quasi-Experiments, and Single-Case Studies, John Wiley & Sons.
- Kramer C.Y. (1957), Extension of multiple range tests to group correlated adjusted means, Biometrics, 13(1).
- Lomax R.G., Hahs-Vaughn D.L. (2012), An Introduction to Statistical Concepts: Third Edition, Routledge.
- Warner R.M. (2013), Applied Statistics: From Bivariate Through Multivariate Techniques, SAGE Publications.
Author: Oksana Szłapowska